Hi students,
I am making this blog for O level students of Physics 5054. I would try my best and post regularly. The whole syllabus of Physics 2014 would be covered here. Do not hesitate to ask away if you are confused about any topic. Furthermore, please do share this blog with your friends if you find it helpful with your studies.
Syllabus Covered So Far
1. Physical Quantities, Units and Measurement
Content
- 1.1 Scalars and vectors
- 1.2 Measurement techniques
- 1.3 Units and symbols
Learning outcomes
Candidates should be able to:
Deformation
6a) state that a force may produce a change in size and shape of a body.
A force can change both the size and shape of a body. This process is called deformation. Though we will mainly study only elastic deformation, this post covers the basics of elastic deformation as well as plastic deformation.
Stretching a spring is a form of elastic deformation. You apply a force, and the size of the spring increases. However, once you stop applying the pulling force, the spring returns to its original shape.
In plastic deformation, an object is permanently damaged, and does not return to its original size or shape even when the force that caused the deformation stops acting on it.A force can change both the size and shape of a body. This process is called deformation. Though we will mainly study only elastic deformation, this post covers the basics of elastic deformation as well as plastic deformation.
- Elastic deformation
Stretching a spring is a form of elastic deformation. You apply a force, and the size of the spring increases. However, once you stop applying the pulling force, the spring returns to its original shape.
- Plastic deformation
Stretching a spring is elastic deformation. However, you might have noticed that if you apply a force too large on the spring, and stretch it too much, the spring is permanently misshaped. Now, it won't return to its original size. This is an example of plastic deformation.
Stability and Center of Mass
(f) describe qualitatively the effect of the position of the center of mass on the stability of simple objects.
As in most occasions, the center of mass and the center of gravity of an object act at the same point, we can consider the effects of center of gravity on the stability of an object.
As in most occasions, the center of mass and the center of gravity of an object act at the same point, we can consider the effects of center of gravity on the stability of an object.
Center of Mass
(e) describe how to determine the position of the center of mass of a plane lamina.
Center of Mass
The center of mass of an object is the point where the average of all the mass of the object is supposed to be concentrated.
Finding Center of Mass of a plane lamina.
Finding the center of mass of a plane lamina is quite easy. Look at the following picture.
To find the center of mass, we will need a plumb line, a clamp stand and a piece of lamina. We will first make a hole anywhere near the edge of the lamina. The lamina will be suspended through the hole in the clamp stand, so that it is hangs and moves freely.
The plumb line would then be suspended from the same point the lamina is hanging. Draw a line on the lamina where the plumb line passes on it. Now we will make another hole at the edge of the lamina, at some distance from the first hole. The process of hanging the lamina, the plumb line and then drawing a line would be repeated.
Now, where the two lines on the paper intersect each other, that point would be the center of mass of the paper.
Verifying The Principle of Moments
(d) Describe how to verify the principle of moments.
We have already covered the principle of moments in the previous post, as well as the principle of moments for a body in equilibrium
To verify the principle of moments, we can use the following experiment.
Experiment:
First of all, we will balance a meter scale on its 50cm mark. Then on the 70 cm mark of the rule, we will put three weights of 4,6 and 10 N respectively.
Now we will calculate the moment due to these three weights. We know that the distance from the pivot is 20 cm. We will first convert it to meters, that is 0.2 m. Now, the moments of these forces will be (4 * 0.2) + (6 * 0.2) + ( 10 * 0.2) which equals to 4 Nm
Now, we will use a weight which is equal to the sum of the previous three weights, that is a weight of 20 N. We will put this weight at the 30 cm mark of the rule, that is the same distance of 20 cm from the pivot, but this time on the other side of the pivot to balance the scale.
The moment produced by this weight 20 * 0.2 = 4 Nm. If the meter rule is uniform, it would balance, therefore verifying the principle of moments, which states that the moments produced due to several forces applied at a single point, are equal to the moment produced by the sum of those forces.
We have already covered the principle of moments in the previous post, as well as the principle of moments for a body in equilibrium
To verify the principle of moments, we can use the following experiment.
Experiment:
First of all, we will balance a meter scale on its 50cm mark. Then on the 70 cm mark of the rule, we will put three weights of 4,6 and 10 N respectively.
Now we will calculate the moment due to these three weights. We know that the distance from the pivot is 20 cm. We will first convert it to meters, that is 0.2 m. Now, the moments of these forces will be (4 * 0.2) + (6 * 0.2) + ( 10 * 0.2) which equals to 4 Nm
Now, we will use a weight which is equal to the sum of the previous three weights, that is a weight of 20 N. We will put this weight at the 30 cm mark of the rule, that is the same distance of 20 cm from the pivot, but this time on the other side of the pivot to balance the scale.
The moment produced by this weight 20 * 0.2 = 4 Nm. If the meter rule is uniform, it would balance, therefore verifying the principle of moments, which states that the moments produced due to several forces applied at a single point, are equal to the moment produced by the sum of those forces.
Calculating Moments & Principle of Moments
(c) make calculations using moment of a force = force × perpendicular distance from the pivot and the principle of moments.
We have already covered that the moment of a force = force applied * perpendicular distance from the pivot. In this post, we will solve a few more questions to further clarify this topic.
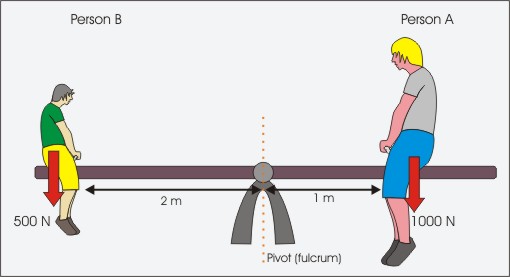
The weight of person A is 1000 N. His distance from the pivot is 1m. Therefore, the moment due to his weight, that is the clockwise moment is equal to force * perpendicular distance from the pivot i.e 1000 * 1
= 1000 Nm.
Person B weighs 500 N. Now, you might think that as person B weighs lesser than person A, the moment due to his weight would be lesser than person A. This can not be decided until we see the distance from the pivot, that is 2 m. Calculating the moment, 500 * 2 = 1000 Nm, we see that the anticlockwise moment due to person B is equal to the clockwise moment due to the weight of person A.
Therefore, we must always keep in mind that force and perpendicular distance from the pivot both play an important role in determining the moment of a force.
We have already covered that the moment of a force = force applied * perpendicular distance from the pivot. In this post, we will solve a few more questions to further clarify this topic.
The weight of person A is 1000 N. His distance from the pivot is 1m. Therefore, the moment due to his weight, that is the clockwise moment is equal to force * perpendicular distance from the pivot i.e 1000 * 1
= 1000 Nm.
Person B weighs 500 N. Now, you might think that as person B weighs lesser than person A, the moment due to his weight would be lesser than person A. This can not be decided until we see the distance from the pivot, that is 2 m. Calculating the moment, 500 * 2 = 1000 Nm, we see that the anticlockwise moment due to person B is equal to the clockwise moment due to the weight of person A.
Therefore, we must always keep in mind that force and perpendicular distance from the pivot both play an important role in determining the moment of a force.
Principle of Moments
The principle of moments state that the total moment produced by several different forces applied at the same point, is equal to the the moment produced by the sum of those forces.
Let's say we apply three forces of 3, 5 and 10 N at a distance of 1 m from the pivot. Now, the moment produced by these forces would be (3 * 1) + (5 * 1) + (10 * 1) = 18 Nm
Now according to the principle of moments, this moment should always be equal to the moment produced by the sum of these forces, if applied at the same point. The sum of these forces is 10 + 5 + 3 = 18 N. Now calculating the moment, 18 * 1 = 18 Nm, which is equal to our value in the first place.
Subscribe to:
Posts (Atom)